Application Notes Optical Components Laser Crystals and Components Infrared Optics Optical Filters Scintillators Wafers and Substrates Crystal Materials Glass Materials

Blog Latest

General Introduction to Optical Coatings

This optical coating guide explores the fundamentals, types, materials, and processes, providing a detailed introduction to optical coatings for anyone seeking knowledge on this critical topic...

Important Specs for Infrared Thermal Imaging Camera Lenses

When it comes to purchasing the proper infrared (IR) thermal imaging camera lens for your specific usage, understanding the technical specifications is crucial to ensure that you acquire the lenses th..

Understanding Optical Material Properties

In this article introducing optical properties, we will be discussing learning several important glossaries of optical material properties including: Refractive Index, Chromatic Dispersion, Optical Tr..

A General Guide to Fisheye Lenses

This article is a general guide and introduction to fisheye lenses, in which the topics about fisheye lenses are discussed, including the characteristic of fisheye lenses, the difference between fishe..

What are Interferomters-Principles, Types, Applications

This is a technical article about interferometers, the content includes an explanation of the light interference phenomenon, the advantages and applications of interferometers, the basic operation pri..

How to Read An Optical Filter Specification Sheet?

What is the optical filter？

Optical filters enable the transmission of specific wavelengths while blocking others across the optical spectrum. Optical filters find versatile utility in applications such as microscopy, chemical analysis, , laser processing, machine vision, medicines, etc. Shalom EO provides a diverse range of optical filters with different precision levels and miscellaneous categories.

This blog elucidates critical optical filter terminologies. We hope this helps you understand these specifications so that you can discern and determine which optical filter best suits your application.

Critical Optical Filter Terminologies

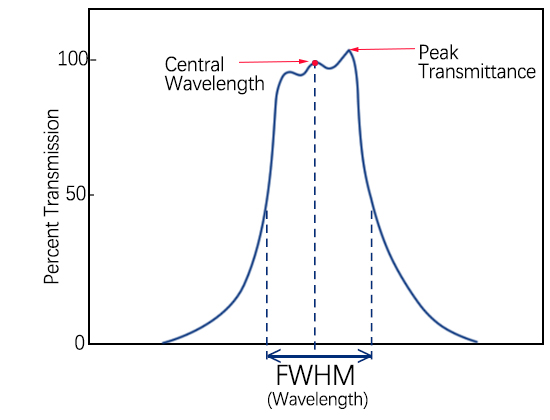
1. Central wavelength:

The central wavelength λc of a bandpass filter can be defined using the formula below:

Where λL, λR correspond to the wavelength positions to the left and right of the passband when the transmittance is half of the peak, respectively. The tolerance of the center wavelength is generally 20% of the bandwidth.

2. Bandwidth：

Bandwidth is the distance between the two positions in the passband where the transmittance is half of the peak transmittance, sometimes called half-height width (not called half bandwidth) often used to indicate FWHM (Full Width at Half Maximum). Specific bandwidth values are defined as follows：

FWHM=λ_R-λ_L

Production tolerances for filter bandwidths are generally based on 20% of the bandwidth size.

3. Peak Transmittance (Peak Transmission Rate(%))

Peak transmittance is the highest transmission rate in the passband of a bandpass filter.

4. Cutoff depth

Cutoff depth is the transmission rate of light allowed to pass through the cutoff band. For different applications, the cutoff depth requirements are different, for example, for the occasions of excitation and fluorescence, the cutoff depth is generally required to be below T < 0.001%. In the ordinary monitoring and identification system, the cutoff depth of T < 0.5% is sometimes sufficient, for occasions with higher demands, the cutoff depth needs to be achieved below T < 0.01%, such as in the application of filters used in the field of autonomous driving.

For simplicity, the cutoff depth is often expressed in terms of optical density (OD), and the relationship between OD and transmittance is as follows: OD=-log10(T) For example, if T<0.01%, the transmittance is 10^-4, which corresponds to OD>4.

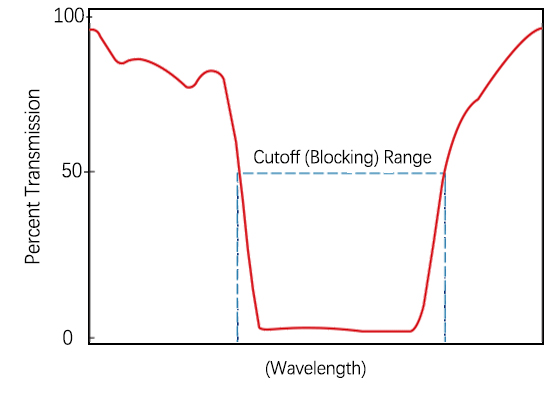
5. Cutoff range (Blocking range)

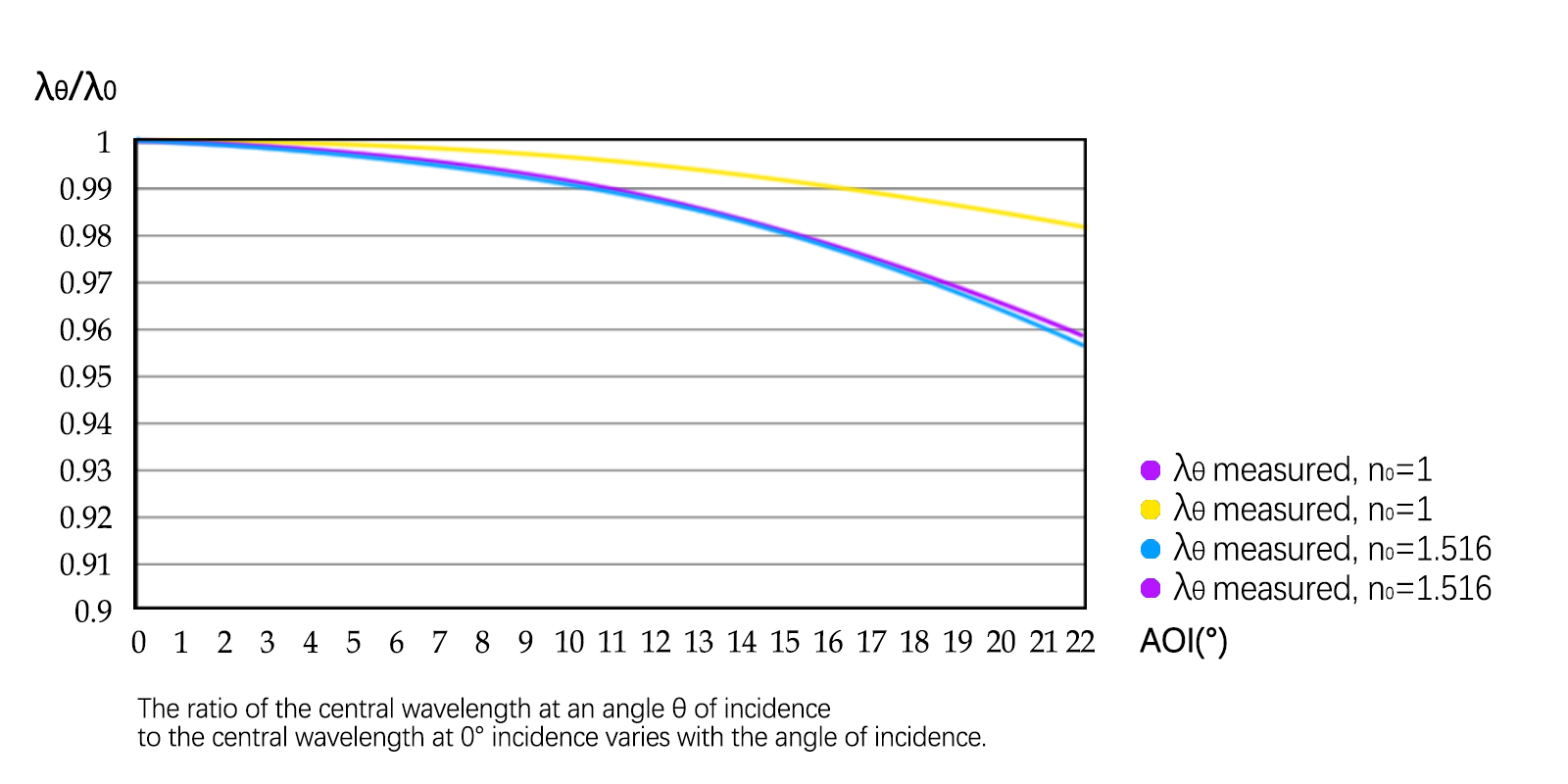
The cutoff range refers to the wavelength range beyond the passband where cutoff is required, apart from the passband itself. For narrowband filters, there are two segments to consider: short cutoff and long cutoff. The short cutoff refers to the wavelength range below the center wavelength where cutoff is required, while the long cutoff refers to the wavelength range above the center wavelength where cutoff is needed. If further granularity is needed, descriptions for both cutoff segments are provided separately. However, in general, specifying the shortest and longest wavelengths where cutoff is required is sufficient to determine the cutoff range for a narrowband filter.

6. The central wavelength varies with the angle of incidence

For narrowband filters, the central wavelength typically varies with the angle of incidence, a phenomenon usually achieved through interference principles. As the angle of incidence increases, the central wavelength of the filter shifts towards shorter wavelengths, and as the angle continues to increase, the rate of short wavelength shift also accelerates. The specific variation follows the following equation:

n₀ is the refractive index of the incident medium surrounding the narrowband filter film. If the narrowband filter film is in the outermost layer, next to the air, then n₀ = 1; if the narrowband filter film is glued in the glass, then the size of n₀ is the refractive index of the glue or glass.

n_eff is the effective refractive index of the narrow band filter film, related to the coatings.

For the sake of visualization, the specific values of the central wavelength shifting towards shorter wavelengths with increasing angle of incidence for two scenarios: n0=1 (narrowband filter system facing air) and n₀=1.516 (narrowband filter system bonded within glass), including both theoretical and experimental values, are plotted in the following figure for reference.

7. Environmental Stability

Shalom EO strives for long-term stability and reliability in its optical filters The coating technology employed, utilizing ion-assisted electron-beam deposition, ensures that the wavelength positions of the filters remain unchanged over time. For deep UV bands below 300nm, such as 220nm, 254nm, 266nm, and 280nm, Shalom EO has adopted a unique sealing technology, which makes the service life of the filters much longer than that of conventional sealing technology.

8. Cutoff Wavelength for Longpass &Shortpass Filters

The cut-off wavelength of the long-pass filter is defined as the wavelength position where the transmittance is 50% (not 50% of the peak value)；The cut-off wavelength of the short-pass filter is defined as the wavelength position point where the transmittance is 50% (not 50% of the peak value).

10. Fluorescent Filter Substrate

For filters used in fluorescent applications, Shalom EO chooses low autofluorescence glass as the coating substrate, such as UV-grade quartz glass or borosilicate glass, e.g., Schott's BF33 glass and Corning's 7980 glass are commonly used as the substrate for fluorescent filters.

Optical Filters