Understanding Optical Material Properties
Overview:
Optical Materials, in the most general sense, are materials that interact with light in various manners, functioning to alter or manipulate electromagnetic radiation ranging from the ultraviolet, visible, and infrared spectral regions. Optical materials are fundamental for a wide range of applications in the field of manufacturing optical elements and optical devices, from simple optical windows and optical lenses to advanced laser systems.
Different optical materials exhibit different properties and understanding Optical Material Properties is crucial because these properties dictate how light behaves when it encounters a material and how the materials react under the impacts of different environments and external forces. It’s almost impossible to enumerate all the material properties, the important ones among the vast sea of optical material property glossaries include transmission, refractive index, absorption and scattering, etc. that indicate the optical characteristics of the materials, in addition to thermal expansion, hardness, strength, that rate the physical stability and durability of optical materials.
Some optical materials are utilized due to their high optical transmission, such as in the case of optical windows, some optical materials are chosen due to their absorptive nature to certain wavelengths of light, such as in the case of optical filters, while some are valued for particular applications due to their special material properties (e.g. materials with high optical nonlinearities are utilized for harmonic generations). By interpreting these properties, engineers and scientists can select the appropriate material for specific applications, ensuring optimal performance in devices such as infrared cameras, microscopes, lasers, etc.
In this article introducing Optical Material Properties, we will be discussing learning several important glossaries of optical material properties including Refractive Index, Chromatic Dispersion, Optical Transmission, Absorption and Light Scattering, Optical Homogeneity, Laser Induced Damage Threshold (LIDT). In the end section, we will also explore the main types of optical materials and their characteristics.
This article is the third of our blog series "Understanding Optical Specifications", in our other two previous blogs about optical specifications, we have also introduced lots of essential glossaries and definitions of optical specifications, which are critical parameters that define the functions and performance of an optic. You can click on the links if you are interested in knowing more about :
1. Optical Geometric Specifications (Dimensional Tolerance, Center Thickness Tolerance, Angle Tolerance, Centering Error, Radius of Curvature, Clear Aperture, etc)
2. Optical Surface Specifications (Surface Quality, Surface Flatness, Surface Roughness, Power, Irregularity, Fracture/Chip).
Refractive Index
The Refractive Index, also named the Index of Refraction of an optical material measures how much light is bent as it enters the optical material. Refraction exists because of the different densities of optical media. Refraction takes place at the interface of two optical media when light travels from one optical medium to another optical medium. The refractive index (n) of a material is defined as the ratio of the speed of light in a vacuum to the speed of light in the material:
n = v/c
Where c is the speed of light in a vacuum, v is the speed of light in the material.
A higher refractive index indicates that light slows down to a greater extent when passing through the material, resulting in greater bending or refraction at the interface between two materials. While a lower refractive index indicates the converse of the above.

Figure 1. Refraction of Light
The refractive index is one of the fundamental optical properties that affects lens design, image quality, and light propagation. For example, materials with a high refractive index can focus light with larger bending angles, allowing for the creation of thinner lenses with the same focal length as thicker lenses made from materials with a lower refractive index.
The refractive index of optical materials varies with the wavelengths of light, and this phenomenon is known as Chromatic Dispersion. The dependence of refractive index on wavelengths can be quantified using the Abbe number or other values such as Group Velocity Dispersion (GVD). The wavelength dependence of the refractive index is also a crucial factor to consider when selecting optical materials. For example, for a femtosecond laser system, optics with a low group velocity dispersion are required because severe broadening optical pulses as the pulses propagate will occur if the refractive index of a material is very wavelength-dependent.
It is also worth noting that some optical materials are birefringent. Due to the optical anisotropy of the material, a birefringent material can have two refractive indices if it is a uniaxial birefringent material or three refractive indices if it is a biaxial birefringent material.
The refractive properties of materials can also change due to changes in surrounding temperatures. Or across the entire material, where the variation in refractive indices is manifested as Optical Inhomogeneity.
Chromatic Dispersion
As mentioned above, Chromatic Dispersion is a phenomenon that occurs when the refractive index of an optical material varies with the wavelength of light. This variation causes different colors (wavelengths) of light to refract, or bend, by different amounts as the light components pass through the material. As long as the light is polychromatic (i.e. light consisting of more than one wavelength), chromatic dispersion is present. From a theoretical viewpoint, for monochromatic light (i.e. light consisting of just one single wavelength), chromatic dispersion does not exist. Since in a strict sense, there’s no genuine monochromatic light with zero optical bandwidth, chromatic dispersion is omnipresent. However, quasi-chromatic light such as laser is often regarded as having only one given wavelength in calculation.
For the most part, the chromatic dispersion of an optical material is represented using the refractive index of the material at three wavelengths: nF at 486.1 nm (Hydrogen F line), nD at 589.3 nm (Yellow Sodium D line), and nC at 656.3 nm (Hydrogen C line).
The Abbe number quantifies this effect, the higher the Abbe number, the lower the dispersion. The Abbe number can be calculated using the formula below:
V = (nD-1)/(nF/nC)
For the application of some optics, chromatic dispersion is detrimental. For instance, one of the most common consequences of chromatic dispersion is Chromatic Aberration (Lerarn more about Chromatic Abberation), a type of distortion where colors are focused at different distances from a lens, resulting in blurred spots on the focal plane and degrading image quality. In some cases, chromatic dispersion is harnessed to separate light into its constituent colors. A dispersive prism, for example, uses dispersion to spread out the spectrum of light. In conclusion, chromatic dispersion is an essential optical property that must be considered when designing optics, particularly those that involve multiple wavelengths of light.

Figure 2. Chromatic Dispersion in a triangular prism. A ray of a white light source is separated into polychromatic lights.
Optical Transmission
Optical Transmission refers to the amount of light that passes through a material without being absorbed or scattered. The inherent properties of the material, including its atomic structure and bonding, determine how much light it can transmit. Optical transmission is quantified using Transmission Rate, which is the ratio of the intensity of the transmitted light to the intensity of the light before entering the material measured in percentage. The transmission rate is defined using the following formula:
Tranmission Rate (%) = (I transmitted/I incident) x 100
Where “I transmitted” is the intensity of light that passes through the material, and “I incident" is the intensity of the incoming light.
This optical transmission property is also wavelength-dependent, meaning that a material might transmit certain wavelengths with higher efficiencies than other wavelengths. For example, germanium has excellent transmission in the IR spectral region, with its transmission range widely spanning from 2μm to 14μm, covering the MWIR and LWIR spectra. However, germanium is opaque to the UV and the visible spectral bands.
Another important point to note is that the transmission rate of optical materials can be significantly enhanced by anti-reflective coatings (anti-reflection coatings), which are often abbreviated as AR coatings. AR coatings are made of materials with suitable optical properties deposited on the exterior optical substrates, minimizing reflection at the material's surface. AR coatings are often dielectric coatings (also known as thin-film coatings or interference coatings). Dielectric coatings can be composed of one single layer or multiple layers, where the interference of reflections from multiple optical interfaces is leveraged to adjust the refractive nature of the surface. The AR coatings are indispensable when manufacturing optical components such as optical lenses, optical windows, and laser crystals, where securing a high light transmission is critical for image clarity or maximizing laser output. The opposite of AR coatings is high-reflection coatings (HR coatings), which are utilized to elevate the reflection rate (reduce the transmission) of the substrate materials. Optical mirrors often use HR coatings.

Figure 3. Transmission Curve of Ge Lens with AR/AR Coating (1mm Thickness Witness Sample)
Besides, there is also a set of factors that might affect the transmission property. The optical homogeneity of the material is another element that we should take into consideration when we look at the transmission rate of optical materials, we will come to more a elaborate discussion of this in the later section of this article. The thickness of the material will also influence the optical transmission of materials, Thicker materials in general have lower transmission rates because light has to travel a longer path, increasing the loss of light intensity. In addition, other properties and parameters like the surface quality of the optical component will also have an impact on its transmission property.
In most cases, a high transmission rate is desirable, for instance, for IR camera lenses, higher transmission contributes to the production of brighter, clearer thermal images; for optical windows, high transmission is imperative to allow light to pass through without significant loss. In some cases, like the optical filters, the transmission rates of the substrate materials and coatings are tailored to achieve the desired filtering effect to select the wanted wavelength bands from the rest.
Absorption and Light Scattering:
Absorption and Light Scattering are two processes that reduce the efficiencies of light transmission through an optical material.
Absorption occurs when the energy of light is absorbed by the material, often converting to heat. The mechanism behind light absorption is that light photons might be absorbed if their energy matches the energy difference between two electronic states in the material's atoms or molecules. The absorbed energy is then dissipated, leading to a decrease in the intensity of transmitted light. The absorption of light in a material is often quantified by the absorption coefficient (α), which indicates how much light is absorbed per unit distance. A high absorption coefficient implies that the material has a greater likelihood to absorb light over a short distance, while a low absorption coefficient indicates that the material has high transparencies. The absorption property of material is very wavelength-dependent, an optical material can be very absorptive to certain wavelengths while very transparent to specific wavelengths.
Light Scattering happens when light is redirected in various directions due to imperfections/irregularities within the material or imperfections on the optical surfaces. The result of light scattering is the deviation of light from its original path. Major factors that determine light scattering include but are not limited to particle sizes, distribution of the particles within the material, or the surface roughness of materials. Scattering is one of the main causes of propagation losses in optical components. Light scattering can be divided into different types, including Rayleigh Scattering, Mie Scattering, Raman Scattering, and Brillouin Scattering. For uses for some materials where propagation losses should be minimized (e.g. ceramic media), the light scattering properties of materials pose a technical predicament. However, light scattering can also be exploited.
Optical Homogeneity:
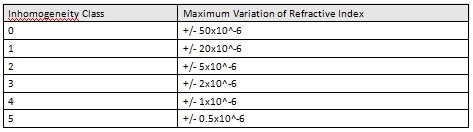
Optical Homogeneity is a material property that indicates the variation of refractive index across the entire volume of the optical materials. A uniform measure to scale optical homogeneity is the Optical Homogeneity Class in the ISO standard. The optical homogeneity is divided into 6 classes from 0-5, each class has a maximum limit of variation of refraction index allowed. High optical homogeneity implies consistent refractive properties of the materials per spatial change inside the material.
The 6 optical homogeneity class and their corresponding maximum variation of refractive index are shown in the table below:
Table of Optical Inhomogeneity Class
Optical Nonlinearity:
Optical Nonlinearity describes the nonlinear behavior in certain optical materials when they interact with light of high intensities. Nonlinear optics is a generalized term for a branch of optics that explores various nonlinear phenomena, such as Harmonic Generation (also known as Optical Frequency Conversion), Pockels effect, Kerr effect, etc.. and the interaction mechanisms behind them are different, giving rise to a set of parameters to characterize the optical nonlinear properties of materials.
Some of the common examples of the applications of optical nonlinearities include harmonic generation (Know more about What is Harmonic Generation) in Nonlinear Crystals, where the X^2 parametric nonlinearity is utilized to change the wavelength of the input signal to the desired wavelength which is not obtainable otherwise; electro-optical modulation utilizing the Kerr effect (derived from the X^3 parametric nonlinearity of the material) and the Pockels effect.
Laser-Induced Damage Threshold (LIDT)
The Laser-Induced Damage Threshold (LIDT) measures a material's largest magnitude of power per area that a material can endure without damage. This property must be paid with significant attention if the materials are to be used in laser systems, where even small amounts of damage can compromise performance. For the most part, laser-grade optics will be specified with their laser damage threshold. For example, look at a Super Achromatic Waveplate from Shalom EO with a damage threshold of 0.5 J/cm^2 (1064 nm, 10 ns, 10 Hz). This means that the super achromatic waveplate can withstand power densities of 0.5J per cm2 from a 1064nm pulsed laser with a pulse duration of 10ns and pulse repetition of 10Hz. Materials with high LIDT, like specialized crystals and glasses, are chosen for high-power laser applications to ensure durability and longevity.
Thermal Properties
Thermal Properties like the Thermal Expansion Coefficient, Temperature Coefficient of Refractive Index, and Thermal Shock Resistance are vital for optical materials used in environments with volatile temperatures.
Thermal Expansion Coefficient (also called Coefficient of Thermal Expansion, CTE) is used to scale the thermal expansion properties of materials. Coefficient of Thermal Expansion, CTE is a generalized term for either one of three parameters of changes in the dimension, area, or volume of optical materials per degree change in temperature under constant pressure. However, in the profession of optics, the prevalent parameter that the suppliers use is Linear CTE, which is the length variation of material in correspondence to temperature changes. Thermal expansion is an important property to consider when choosing optical materials. Materials with low thermal expansion, such as certain ceramics and optical glasses, maintain their shape and optical performance under temperature changes.
Another prior thermal properties of optical materials is the Temperature Coefficient of Refractive Index (dn/dT). The temperature coefficient of refractive index (dn/dT) defines how the refractive index changes via temperature changes. The dn/dT measure is vital in some particular applications, for example, for IR-transmitting materials, the temperature coefficient of refractive index is far greater than common visible glass, therefore when designing IR lens assemblies for thermal imaging cameras, this parameter must be taken with cognition, the change in refractive index resulted from temperature change can cause thermal defocus of the lenses. One measure to alleviate the problem of thermal defocus is Optical Passive Athermalization, where different lens materials with opposite dn/dT properties are configured together to cancel out the variation in refractive index due to temperatures.
Thermal Shock Resistance refers to the ability of a material to withstand rapid changes in temperature without cracking or breaking. Thermal shock resistance is of great concern for materials exposed to rapid temperature fluctuations, as it prevents fracture and deformation.
Mechanical Properties
The Mechanical Properties of optical materials, including Hardness, Strength, Elasticity, etc. serve a significant role in determining their durability and suitability for various applications.
Hardness is the resistance of a material to localized plastic deformation. Hard materials resist scratches and abrasions. The hardness of optical materials is often rated using the Mohs hardness, where a higher value indicates greater hardness.
Strength can divided into mechanical strength, the ability to withstand an applied stress or load without structural failure; compressive strength, the capacity to withstand pushing forces in axial directions. Tensile strength is the maximum stress while being stretched or pulled before necking. Strong materials withstand mechanical stresses during manufacturing and operation. Strength shouldn’t be confused with hardness, an optical material described as being hard might not necessarily be strong at the same time.
Elasticity allows materials to flex under stress without cracking, which is important in dynamic environments.
Different Types of Optical Materials:
There are numerous optical materials, and in general optical materials can be classified into four major types according to the microscopic structures of the materials: crystalline materials, inorganic glass, ceramics, metals, and organic polymers. Each type of optical material has its appealing attributes and disadvantages, for example, optical glass materials stand out for their excellent transparencies to light, the simple fabrication process, and the large flexiblities to modify their properties. However, the drawback of optical glasses is that they tend to be vulnerable. The distinctive pros and cons of each type, in combination with the cost factor, determine what type should be used for manufacturing optical elements.
1. Inorganic Glass
Glass is an inorganic solid material of amorphous (non-crystalline) state. Glass is one of the most common optical materials, known for its excellent optical transparency, durability, and ease of fabrication. Glass also tends to be cheaper than crystalline materials due to its abundant availability. Different types of optical glass are engineered to offer specific properties such as maximized transmission and minimal dispersion. Common applications of optical glass include lenses, prisms, and optical fibers. For instance, crown glass has a lower refractive index and dispersion, making it ideal for general-purpose lenses, while flint glass has a higher refractive index and is advantageous for applications like lenses. Common optical glass materials include N-BK7, Fused Silica, etc.
2. Crystals
Crystalline Materials are solid materials whose constituents (like atoms, molecules, or ions) are arranged in ordered microscopic structures. Most optical crystals are single crystals to avoid the scattering introduced by the grain boundaries. Crystals are sometimes used in the replacement of glass material to achieve higher transmission, e.g. for mid-IR to far-IR spectra, crystals are often chosen instead of glass to gain better transparencies.
In most cases, the regular and consistent arrangements in the microscopic structures of crystals are exploited to gain certain effects, for example, to manipulate the polarization state of light. Nonlinear crystals with high levels of asymmetry and exhibiting a strong nonlinear dielectric response function to optical radiation can be used for various Nonlinear Optical (NLO) applications. Crystals can also be doped with laser-active ions to generate lasers of different wavelengths.
3. Organic Polymers
Organic Polymers, sometimes also called Organic Glass, are optical materials consisting of repeating monomers. Typical examples of organic polymers include polypropylene, polyethylene, polyvinyl chloride (PVC), nylon, polystyrene, etc. With the attractive properties of lightweight, versatile, low cost, and being molded into complex shapes, organic polymers become attractive candidates for the production of aspheric optics (learn more about aspheric optics) and dyed optical filters. However, the disadvantage of organic polymers is that the polymers tend to have lower optical quality than glass and are susceptible to high temperatures.
4. Metals
While not transparent, Metals serve an essential role in optical systems due to their reflective properties. Metals like aluminum, silver, and gold are used in mirrors, coatings, and reflective optics. Silver, for instance, has one of the highest reflectance across the visible spectrum and is often used in high-performance mirrors. Aluminum is suitable for lightweight and cost-effective reflective coatings.
5. Polycrystalline Ceramics
Polycrystalline Ceramics are materials comprised of grains with irregular crystalline orientations. Over the years, ceramics have seen rapid growth in manufacturing optics due to their hardness, malleable properties via modifications, and resistance to extreme conditions. Optical ceramic material like Yttrium Aluminum Garnet (YAG) doped with Nd ion is great when adopted as laser gain media with similar properties to single crystal Nd: YAG but better cost-effectiveness. In general, ceramic materials exhibit excellent properties to withstand high temperatures, thermal shock, and mechanical stress, making them suitable for challenging optical applications like thermal imaging. The main technical stranglehold for the application of ceramic materials optics is the light scattering taking place at the grain boundaries, however, up to now, some ceramic materials like alumina (aluminum oxide) or YAG have been improved so that the light scattering is less significant.
You might also be interested in:
Optical Basics: The Nature of Light and Optical Elements
Tips: The understanding of the various glossaries of optical material properties requires the comprehension of some basic concepts about light, such as wavelenth, refraction, etc. Reading the resource above might be helpful.
Conclusion
A comprehensive understanding of optical material properties is essential for the successful design and application of optics. Whether you're dealing with lenses, windows, or laser components, knowing the refractive index, optical transmission, and thermal properties, etc. of a material will help you make rational choices that enhance performance and reliability. This introduction to optical material properties serves as a foundation for exploring the vast possibilities in the world of optics, where material selection is key to innovation and success.
Related Articles
Tags: Understanding Optical Material Properties